A story about quasiparticles

Imagine, if you will, that you are an alien from some advanced and distant civilization.  You find yourself fascinated by humans, whom you observe from your own planet through an ultra-high-powered telescope.  As individuals, you think you know what humans are like: at least you have a sense of their characteristic size and patterns of motion.  But you are puzzled by the behavior of large groups of humans.  You therefore decide to make a study entitled “the properties of large, densely-packed groups of humans”.  You begin your study by turning the gaze of your telescope to the biggest, densest group of humans you can find: the crowd at a football stadium.

The collection of humans inside the football stadium seems at first to be an enormous, chaotic, impossibly-complex collection of individual movements.  But after a long period of observation, you see something truly remarkable: the humans begin doing “the wave”.  What a startling observation this would be!  From 80,000 humans packed together and moving around in a hopelessly complicated mess arises something remarkably simple: a single wave, which moves around the stadium with its own characteristic size and speed.  You complete your study by observing “the wave”, writing down laws that describe its size and speed, and trying to predict when and where it will occur in the future.

The reason I tell this funny story is to make the following point: sometimes doing physics is like being an alien and watching “the wave”.  In physics we examine large collections of objects, which we think we understand individually, and try to make sense of what they are doing collectively.  If you’re a scientist, and you get to observe the emergence of a simple and unexpected thing from the complex group, then that’s a beautiful moment.  And if you can somehow understand how it is that all the individual objects created, in concert, the simple emergent thing, then that’s a really beautiful moment.  In physics we live for beautiful moments.

Unfortunately, the language physicists use to describe things is usually pretty boring (see the Calvin and Hobbes comic below), so a lot of the great stories in physics sound a lot more boring than they are.  A surprisingly simple, emergent thing that arises from the collective interactions of a huge group of individual things usually gets called a “quasiparticle”.  It’s a boring name that describes a fairly fascinating concept: a quasiparticle is an object that moves and responds as if it were its own independent particle, even though it is really the result of the collective motion of lots of individuals.  Identifying and describing quasiparticles is one of the more fun things that physicists do.

In this post I want to tell the story of a particular kind of quasiparticle: a dislocation in a two-dimensional electron gas.  It’s a particularly satisfying story, I think, because it can be understood and described without a lot of complicated math, and because it leads directly to an audacious line of imaginative further questions.

The field

A common theme among quasiparticles is that they arise from a large “field” of constantly shifting, jostling individuals.  “The wave” at a football game, for example, was made from the “field” of people packed inside the stadium.  For the quasiparticles I want to describe now, the field is what we call a two-dimensional electron gas.  Basically, you can think of it as a bunch of charged balls (electrons) which slide around on top of a large, flat plate of opposite charge.  Like this:

 

Cartoon version of a two-dimensional electron gas: negative electrons (blue) sit on top of a positively charged plate (red).

Individual electrons are attracted to the plate, but are repelled from each other.  As a result, they try to space themselves out evenly in order to neutralize the plate.  That way that there are no regions of the plate with too much positive charge (a scarcity of electrons) or too much negative charge (electrons crowded together).  Of course, unless the electron gas is at absolute zero, the electrons will have some thermal energy and will  jostle back and forth a little bit as they slide around on top of the positive plate.  If you start cooling everything down, though, and thereby rob the electrons of their thermal motion, the electrons slowly settle into an organized and very regular configuration.  They take on the form of a hexagonal (or honeycomb) lattice.  Like this:

This picture, and the next few, are adapted from an image at chem.purdue.edu (click the picture). Please excuse the graininess.

This honeycomb structure is the arrangement that keeps the electrons furthest separated from each other while still maintaining the density required to neutralize the positive plate.  It is the perfect balance between the electrons’ attraction to the positive plate and their repulsion from each other.  We call this arrangement the “ground state”; it minimizes the total energy of the system.  It’s funny how something with six-fold symmetry can arise spontaneously from the spherically-symmetric force between electrons.  But there it is.

You may notice that the electrons in the lattice above are arranged in orderly, parallel lines which run in three directions: horizontally, slanted to the left, and slanted to the right.  Here is that same lattice, with the lines of symmetry shown in red:

The orderliness of the lattice can be characterized by how regular these “lattice lines” are: whether they maintain their spacing and orientation over long distances.

Defects in the field

Now that you know what a perfectly-ordered electron gas looks, you can ask what happens when we add back a little thermal energy.  Naturally, the electrons start kicking around again and they lose their perfectly ordered structure.  In other words, we start to see regions of higher- and lower-than-average electron density.  Something like this, for example:

If you look closely, you’ll see that the area in the center of this figure has a little hole in it.  That is, the jostling of individual electrons has created a region where the electrons are more sparse than would be expected.  This disrupts the lattice lines.  If you try to draw them all out, you’ll find that one of the lines (going down to the right) ends right at the defect region.  Like this:

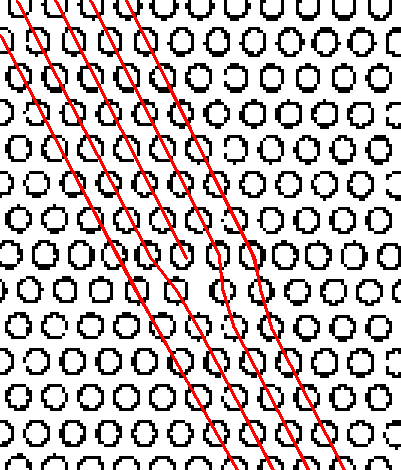
This defect is called a “dislocation”.  It is a spot where one of the lattice lines comes to an end.  And since the electrons are all moving about, the dislocation is not fixed in space; it can move around as individual electrons shift.  Imagine, for example, if the electron immediately to the right of the dislocation moved a little to the left; this would complete the broken lattice line but break the one immediately to the right, thereby moving the dislocation one space to the right.  In this way the dislocation can glide around the field of electrons, just like “the wave” moves through a stadium.  The random, individual motions of electrons will send the dislocation moving randomly around through the field.  The dislocation is  destroyed only when it comes into contact with another dislocation pointing the opposite direction, i.e. when two broken planes meet each other at their loose ends.  And in fact, dislocations arise from the ground state only in opposite pairs.

Quasielectrons and quasipositrons

You can see now why a dislocation is a little bit like a real particle: it arises as part of a spontaneously-created pair and glides randomly around the field until it annihilates (peacefully) with an opposite.  But the truly surprising “particleness” comes from the way in which it attracts and repels other dislocations.

A dislocation creates strain in the field around it.  The electrons immediately surrounding the dislocation are highly strained: they get pushed into each other or rarified from one another in a way that costs energy.  Electrons farther from the dislocation are only mildly strained, and the amount of strain in the field decays with distance.  There is actually a neat way of figuring out the degree of strain in the field as a function of distance that is very similar to Gauss’s Law:

 

A path that would normally be closed will fail to return to itself by one "unit" if it encloses a dislocation. So the perimeter of each closed loop shares "one unit" of strain. Image taken from this paper: http://prola.aps.org/abstract/RMP/v60/i1/p161_1

I won’t go too far into the specifics, but the upshot is that the “strain field” surrounding a dislocation is very much like the electric field that surrounds a real, two-dimensional electron.  When two same-direction dislocations are brought close to each other, the strain in the field becomes very large, and as a result the dislocations are pushed apart.  If two opposite dislocations are brought close to each other, the strain in the field pulls them together.

It’s worth pausing here to emphasize the real point of this post: just like “the wave”, the emergence of these quasiparticles would be a surprising and beautiful thing to observe.  Imagine it like this: you decide to study a complicated jumble of electrons, which you watch through your high-powered measurement devices.  After observing for a while, you see something remarkable: a “defect” arises in the field of electrons, which travels around with its own characteristic size and speed.  Even more astonishing, this defect behaves as if it were a real electron (call it a “quasielectron”).  It repels other quasielectrons in exactly the same way that real electrons repel each other, and it attracts “quasipositrons” (opposite-facing dislocations).  The two can even annihilate each other, or arise spontaneously from the field, just like real electrons and positrons do.

I should mention, as a side note, that the main features of this story would be completely unchanged if we replaced the electrons with something else.  As long as we have a collection of objects that are repelled by each other and attracted to the space they sit on, the same sorts of quasiparticles will arise.  There would still be a hexagonal lattice, with opposite-facing dislocations that appear spontaneously, and a “Gauss’s Law” analogy that determines how dislocations strain the field around them.  In other words, the existence of “quasielectrons” and “quasipositrons” does not require the existence of real electrons and positrons.

Impudent Questions

It only takes a little bit of impudence to hear this story and want to ask the following question: what if real electrons actually are “quasiparticles” in some field that’s too small to see?  What if an electron is really some kind of defect in a vast, space-filling lattice of tiny, jostling objects?  What if all the matter and energy we observe in the universe is really a collection of defects and ripples propagating through the cosmic “fabric”?

If the startling similarities between real particles and quasiparticles are enough to convince you that these “cosmic fabric” ideas must be true, then that makes you a crank.  But if this story doesn’t get your imagination going, then I don’t know what to tell you.  Because these are the beautiful moments that physicists live for.

I like to think that a story like this one must have been the inspiration for quantum field theory, which describes all matter as a disturbance in some space-filling field.  Outlandish as it may sound, it is arguably the most successful physical theory we have.  I only wish that my quantum field theory class hadn’t looked like this all the time.